High-Low Method Calculator

Enter the high and low parameters in the high-low method calculator below:

The high-low method calculator is an important tool used to estimate fixed and variable costs based on limited historical data. This simple calculator can provide valuable insights into cost behavior to improve budgeting and decision-making.

What is the High-Low Method?

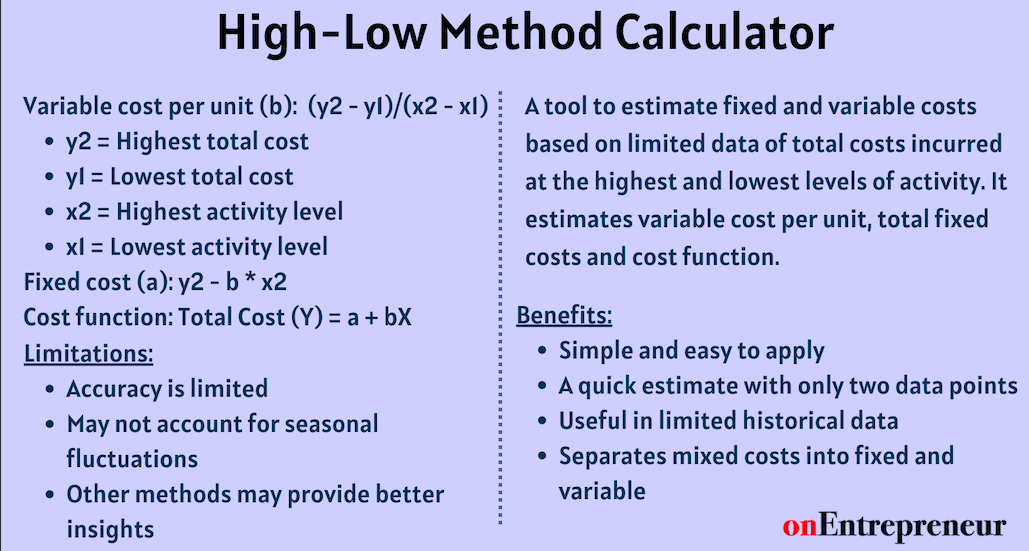
The high-low method is an important cost accounting technique used to estimate fixed and variable costs based on limited historical data. It involves comparing the total costs incurred at the highest and lowest levels of activity. By examining the extreme high and low data points, it estimates:

• Variable cost per unit
• Total fixed costs
• Cost function to calculate total cost at any activity level

This method is ideal when there is limited data available. It provides a quick way to determine fixed vs variable costs and understand cost behavior.

How to Calculate the High-Low Method

Here are the steps to apply the high-low method:

1. Identify the highest and lowest activity levels and their associated total costs.
2. Calculate variable cost per unit (b): (y2 – y1)/(x2 – x1)
   • y2 = Highest total cost
   • y1 = Lowest total cost
   • x2 = Highest activity level
   • x1 = Lowest activity level
3. Determine fixed cost (a): Highest total cost – (Variable cost per unit * Highest activity level)
4. Create a cost function: Total Cost (Y)= Fixed Cost (a) + Variable Cost per unit (b) * Units of activity(Y)

This cost function can then estimate total costs for any activity level.

To make it easy, you can use this High-Low Method Calculator above:

Key Benefits of Using the High-Low Method

• Simple and easy to apply without statistical analysis
• Provides quick estimate of cost behavior
• Requires only two data points
• Useful when limited historical data is available
• Helps separate mixed costs into fixed and variable elements

Limitations to Consider

• Accuracy is limited due to only using two data points
• Can oversimplify the cost-volume relationship
• May not account for seasonal fluctuations, inflation changes, etc.
• Other methods like regression analysis may provide better insights

So the high-low method provides a straightforward starting point for cost analysis, but should be complemented with other techniques where feasible.

High-Low Method Example

Here is an example to demonstrate using the high-low method in practice:

Highest activity level: 100 units, $50 total cost

Lowest activity level: 20 units, $30 total cost

Variable cost per unit = ($50- $30) / (100 – 20 units) = $0.25 per unit

Fixed cost = $50 – (100 * $0.25) = $25

Total cost function = $25 fixed cost + $0.25 per unit variable cost

This function allows estimating the total cost for any number of units. You can use the above high-low method calculator to validate this result.

Key Takeaways

• The high-low method compares costs at maximum and minimum activity levels
• It estimates the variable and fixed cost elements
• This simple method is useful when limited data is available
• The resulting cost function provides an approximate cost-volume model
• Use the high-low calculator to easily apply this method

Using this technique can provide valuable fixed vs variable cost insights, but should be combined with other methods for greater accuracy.

Frequently Asked Questions

What are the main limitations of the high-low method?

The main limitations are it only uses two data points, can oversimplify cost behavior, and may not account for seasonal or inflation changes.

When is the high-low method most appropriate to use?

It is most appropriate when limited historical cost data is available. The high-low method provides a quick estimate of cost behavior.

Can the high-low method work with more than two data points?

Yes, you can apply the method using the highest and lowest activity levels from any available data set. Using only the extremes helps simplify the calculation.

How accurate are the results from the high-low method?

The cost estimates tend to be approximate since it relies on two data points. For greater accuracy, combine it with other techniques like regression analysis.

Does the high-low method work for both manufacturing and service businesses?

Yes, the high-low method can be applied to analyze costs for both manufacturing and service companies, using appropriate activity measures like units produced or labor hours.